The day with the least hours of daylight, the "shortest day", usually occurs around the 20th or 21st of December along with the winter solstice. It is often assumed that there is more daylight at both ends of the day after this day, but this is not quite the case. In fact the earliest sunset occurs some days before the shortest day and the lastest sunrise a few days afterwards. Typically, when we come back to work after the Christmas and New Year holiday, the sunrises are later than before the holiday and not much change is visible until well into January.
This plot shows the effect (for 2005 as an example):
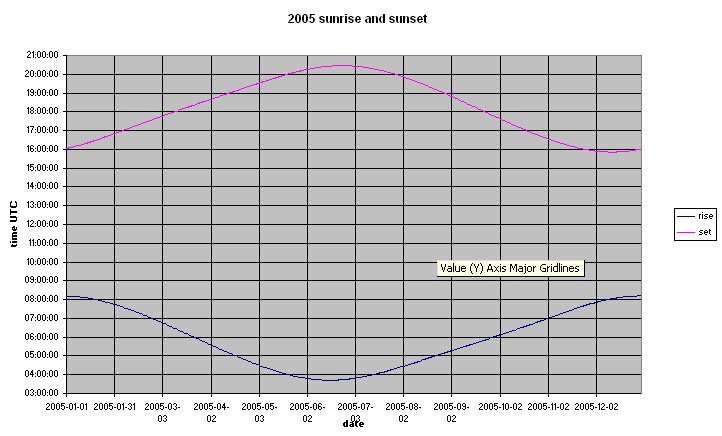
The top curve shows the time of sunset through the year and the bottom line the time of sunrise. Note that at mid summer, the centre part of the plot, the peak for sunset occurs after the trough for sunrise and at mid winter, off one end of the chart and back to the start, the trough for sunset occurs before the peak for sunrise. This also means that in spring the sunrise times change faster day by day than sunset and in autumn the sunset times change faster than sunrise.
This odd behaviour is not a fundamental feature of the movement of the planets. It would not have been obvious to anyone more than a few hundred years ago. It is entirely a product of our dependence on mechanical clocks.
The earth spins on its axis at a roughly constant rate, but what we call a day is slightly more than one turn, as the earth has moved a little further along its orbit around the sun during the day, it has to turn a little further to aim the same spot on earth back at the sun. So, again approximately, a day is about 11/365 of one turn of the earth on its axis. This is what we call 24 hours.
As the earth's orbit is not a circle, and there are other small influences from other planets, the little bit extra needed each day is not always the same. In a solar-driven world, when time is told from a sundial, this effect is invisible. Noon is defined as the time when the sun reaches its highest point and the rest of the day scaled each side. When clocks became good enough to measure this variation the definition of time was changed to be the clock rather than the sun. The definition was chosen to make the clock and the sun agree on average over the course of a year. These days, now that our atomic clocks are very accurate and stable, it is found that this average does not quite fit, there are random variations which need to be corrected by occasional leap seconds.
Once clock time is used to regulate life, the time on a sundial is no longer the "right" time. During the year, it can be as much as 15 minutes fast or slow compared to clock time. The difference between sundial time and clock time is called the Equation of Time, and it is sometimes seen engraved on sundials so that the right time can be estimated. This is a plot of the equation, taken from Wikipedia; it shows the number of minutes by which a sundial is fast compared to a clock:
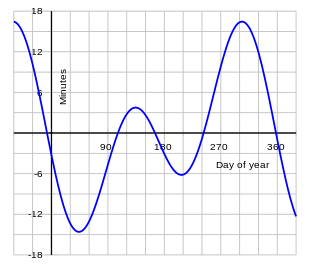
It can be seen that at the turn of the year, near the origin, the difference between the sundial and the clock is changing very rapidly. This is the key to the sunrise and sunset time behaviour.
In fact, sunrise and sunset are symmetrical about noon, the highest sun position, as you would intuitively expect. What is happening is that the clock time at which the sun is highest is changing quickly, more quickly than the sunrise and sunset times are changing as they reach the level parts of the curve where they change direction. This can be seen by doing some calculations.
The table below attempts to do this. For 22 days around the solstice (2011 being used as an example this time) it takes the predicted sunrise and sunset times given in the published tables and adds the equation of time to get back to the equivalent sundial time. The equation of time value is in the column "deltaTa". The published clock sunrise time is in the column "sr UTC" and the sunset time in "ss UTC". The calculated sundial sunrise time is in "sr dial" and sunset in "ss dial". The clock time latest sunrise is highlighted, it occurs on 2011-12-30, and the earliest sunset on 2011-12-13. In the sundial times, the earliest sunset is the evening of 2011-12-21 and the latest sunrise the morning of the next day. The longest day can be seen from the "length" column, it's on 2011-12-22, the same day as the latest sunrise.
So indeed, relative to the sun, sunrise and sunset are symmetrical, and change direction at the same time, on the longest day. The strange behaviour of the published times is due to the clock time of the real "noon" changing by nearly 10 minutes over the interval of 21 days.
| date | d# | n | M | deltaTa | sr UTC | ss UTC | sr dial | ss dial | mid-point | length | mid-point UTC |
| 2011-12-10 | 343 | 341 | 5.866 | 6.729 | 07:59:48.03 | 15:51:36.97 | 08:06:31.76 | 15:58:20.70 | 12:02:26.23 | 07:51:48.94 | 11:55:42.50 |
| 2011-12-11 | 344 | 342 | 5.883 | 6.290 | 08:00:52.31 | 15:51:28.21 | 08:07:09.73 | 15:57:45.63 | 12:02:27.68 | 07:50:35.90 | 11:56:10.26 |
| 2011-12-12 | 345 | 343 | 5.901 | 5.847 | 08:01:53.90 | 15:51:22.93 | 08:07:44.72 | 15:57:13.75 | 12:02:29.24 | 07:49:29.03 | 11:56:38.42 |
| 2011-12-13 | 346 | 344 | 5.918 | 5.399 | 08:02:52.73 | 15:51:21.16 | 08:08:16.69 | 15:56:45.12 | 12:02:30.90 | 07:48:28.43 | 11:57:06.95 |
| 2011-12-14 | 347 | 345 | 5.935 | 4.948 | 08:03:48.72 | 15:51:22.90 | 08:08:45.58 | 15:56:19.76 | 12:02:32.67 | 07:47:34.18 | 11:57:35.81 |
| 2011-12-15 | 348 | 346 | 5.952 | 4.493 | 08:04:41.82 | 15:51:28.16 | 08:09:11.37 | 15:55:57.71 | 12:02:34.54 | 07:46:46.34 | 11:58:04.99 |
| 2011-12-16 | 349 | 347 | 5.969 | 4.034 | 08:05:31.96 | 15:51:36.94 | 08:09:34.01 | 15:55:38.99 | 12:02:36.50 | 07:46:04.98 | 11:58:34.45 |
| 2011-12-17 | 350 | 348 | 5.987 | 3.573 | 08:06:19.08 | 15:51:49.24 | 08:09:53.48 | 15:55:23.64 | 12:02:38.56 | 07:45:30.16 | 11:59:04.16 |
| 2011-12-18 | 351 | 349 | 6.004 | 3.110 | 08:07:03.12 | 15:52:05.06 | 08:10:09.72 | 15:55:11.66 | 12:02:40.69 | 07:45:01.94 | 11:59:34.09 |
| 2011-12-19 | 352 | 350 | 6.021 | 2.645 | 08:07:44.02 | 15:52:24.38 | 08:10:22.72 | 15:55:03.08 | 12:02:42.90 | 07:44:40.36 | 12:00:04.20 |
| 2011-12-20 | 353 | 351 | 6.038 | 2.179 | 08:08:21.74 | 15:52:47.19 | 08:10:32.46 | 15:54:57.91 | 12:02:45.19 | 07:44:25.45 | 12:00:34.47 |
| 2011-12-21 | 354 | 352 | 6.055 | 1.711 | 08:08:56.24 | 15:53:13.46 | 08:10:38.92 | 15:54:56.14 | 12:02:47.53 | 07:44:17.22 | 12:01:04.85 |
| 2011-12-22 | 355 | 353 | 6.073 | 1.244 | 08:09:27.46 | 15:53:43.16 | 08:10:42.07 | 15:54:57.77 | 12:02:49.92 | 07:44:15.70 | 12:01:35.31 |
| 2011-12-23 | 356 | 354 | 6.090 | 0.776 | 08:09:55.37 | 15:54:16.26 | 08:10:41.91 | 15:55:02.80 | 12:02:52.36 | 07:44:20.89 | 12:02:05.81 |
| 2011-12-24 | 357 | 355 | 6.107 | 0.308 | 08:10:19.93 | 15:54:52.71 | 08:10:38.43 | 15:55:11.21 | 12:02:54.82 | 07:44:32.78 | 12:02:36.32 |
| 2011-12-25 | 358 | 356 | 6.124 | -0.158 | 08:10:41.10 | 15:55:32.46 | 08:10:31.60 | 15:55:22.96 | 12:02:57.28 | 07:44:51.36 | 12:03:06.78 |
| 2011-12-26 | 359 | 357 | 6.141 | -0.624 | 08:10:58.86 | 15:56:15.46 | 08:10:21.44 | 15:55:38.04 | 12:02:59.74 | 07:45:16.60 | 12:03:37.16 |
| 2011-12-27 | 360 | 358 | 6.159 | -1.087 | 08:11:13.19 | 15:57:01.66 | 08:10:07.95 | 15:55:56.42 | 12:03:02.18 | 07:45:48.47 | 12:04:07.42 |
| 2011-12-28 | 361 | 359 | 6.176 | -1.549 | 08:11:24.05 | 15:57:51.00 | 08:09:51.11 | 15:56:18.06 | 12:03:04.59 | 07:46:26.95 | 12:04:37.52 |
| 2011-12-29 | 362 | 360 | 6.193 | -2.008 | 08:11:31.45 | 15:58:43.42 | 08:09:30.97 | 15:56:42.94 | 12:03:06.96 | 07:47:11.97 | 12:05:07.43 |
| 2011-12-30 | 363 | 361 | 6.210 | -2.464 | 08:11:35.36 | 15:59:38.85 | 08:09:07.52 | 15:57:11.01 | 12:03:09.27 | 07:48:03.49 | 12:05:37.10 |
| 2011-12-31 | 364 | 362 | 6.227 | -2.916 | 08:11:35.79 | 16:00:37.22 | 08:08:40.80 | 15:57:42.23 | 12:03:11.52 | 07:49:01.43 | 12:06:06.51 |
nib 2012-12-20